In the given figure, O is the center of the circle and TP is the tangent to the circle from an external point T. If ∠PBT = 30°, prove that BA : AT = 2 : 1.
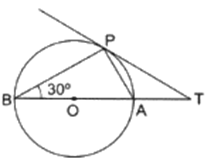
In Given Figure, we have a circle with center O let the radius of circle be r.
Construction : Join OP

Now, In △APB
∠ABP = 30°
∠APB = 90°
[Angle in a semicircle is a right angle]
By angle sum Property of triangle,
∠ABP + ∠APB + ∠PAB = 180
30° + 90° + ∠PAB = 180
∠PAB = 60°
OP = OA = r [radii]
∠PAB = ∠OPA = 60°
[Angles opposite to equal sides are equal]
By angle sum Property of triangle
∠OPA + ∠OAP + ∠AOP = 180°
60° + ∠PAB + ∠AOP = 180
60 + 60 + ∠AOP = 180
∠AOP = 60°
As all angles of △OPA equals to 60°, △OPA is an equilateral triangle
So, we have, OP = OA = PA = r [1]
∠OPT = 90°
[Tangent at any point on the circle is perpendicular to the radius through point of contact]
∠OPA + ∠APT = 90
60 + ∠APT = 90
∠APT = 30°
Also,
∠PAB + ∠PAT = 180° [Linear pair]
60° + ∠PAT = 180°
∠PAT = 120°
In △APT
∠APT + ∠PAT + ∠PTA = 180°
30° + 120° + ∠PTA = 180°
∠PTA = 30°
So,
We have
∠APT = ∠PTA = 30°
AT = PA
[Sides opposite to equal angles are equal]
AT = r [From 1] [2]
Now,
AB = OA + OB = r + r = 2r [3]
From [2] and [3]
AB : AT = 2r : r = 2 : 1
Hence Proved !