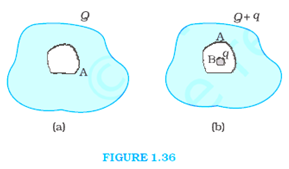
(a) A conductor A with a cavity as shown in Fig. 1.36(a) is given a charge Q. Show that the entire charge must appear on the outer surface of the conductor.
(b) Another conductor B with charge q is inserted into the cavity keeping B insulated from A. Show that the total charge on the outside surface of A is Q + q [Fig. 1.36(b)].
(c) A sensitive instrument is to be shielded from the strong electrostatic fields in its environment. Suggest a possible way.
In Electrostatic we deal with charges at rest so there is no current inside or on the surface of the conductor i.e. in the static situation, the electric field is zero everywhere inside the conductor. This is the basic property of a conductor. A conductor has free electrons. As long as electric field is not zero, the free charge carriers would experience force and drift and re distribute themselves in such a way so that net electric field inside a conductor is zero.
We will use the above stated property to solve the problems
(a) Here in this situation a conductor with a cavity having no charge inside is given a charge Q on outer surface, we have to show that all charge resides on the external surface only and there is no charge on the inner surface.
From Gauss Theorem we know, net electric flux through an Gaussian surface enclosing a charge is equal to net charge enclosed by surface divided by permittivity of free space
Mathematically 
Where E is the electric field so 
denotes the net Electric flux through the surface q is the net charge enclosed by the Gaussian surface and 
is permittivity of free space which is a constant
Now if electric field at all the points on a Gaussian surface is zero
i.e. flux is zero or 
this means q/ϵ0 = 0 or q = 0
i.e if electric field at all the points on a Gaussian surface is zero then the net charge enclosed by the Gaussian surface is also zero, or there is no charge inside the Gaussian surface
Now if we consider a Gaussian surface enclosing the cavity inside the conductor as shown in the figure
we know that net electric field everywhere inside a conductor is zero
so the electric flux through this Gaussian surface would also be zero, this means no charge is enclosed by the Gaussian surface, since cavity does not contain any charge so no charge should be induced on the cavity’s metal surface as well so whole of the charge Q is distributed on the outer surface only.
(b) Again using Gauss Theorem we know,
If electric field at all the points on a Gaussian surface is zero then the net charge enclosed by the Gaussian surface is also zero, or there is no charge inside the Gaussian surface
Now if we consider a Gaussian surface enclosing the cavity inside the conductor as shown in the figure
we know that net electric field everywhere inside a conductor is zero
so the electric flux through this Gaussian surface would also be zero, this means no charge is enclosed by the Gaussian surface, but the cavity has a charge q , this means a charge –q must be induced on the inner metal surface because net charge enclosed by Gaussian surface must be zero (q + (-q) = 0) , but the metal surface has only been given charge Q so net charge of system must only be q + Q , i.e. a charge q is induced on outer surface of the conductor as which makes the total charge on the conductor equal to Q only Q + (-q) + q = Q
and total charge of the system as
Q + (-q) + q + q = Q + q
This arrangement is also in accordance with the law of conservation of charge
note: Only Q charge has been given on the conductor’s outer surface , the charge appearing (Q + q) on it is only due to induction due to charge inside the cavity.
(c) To shield the sensitive instrument from strong electrostatic fields in its environment. We need to enclose it inside a metal piece’s Cavity, this process is also known as electrostatic shielding.
we consider a metal Block with a cavity having no charge inside and Instrument inside it as shown in the figure
any cavity inside a metal surface the electric field is zero no matter in which environment it is placed.
So in order to save the Instrument from External Electrostatic fiels we need to keep it inside a metal Cavity.