The surface areas of two sphere are in the ratio of 4:25. Find the ratio of their volumes.

Let suppose the radius of first spheres = r

And the radius of second spheres = R

As per the question,

Surface area of the first sphere /Surface area of the second sphere = 4/25

⇒ 4πr²/4πR² = 4/25

⇒ (r/R)² = 4/25

⇒ r/R = √4/25

⇒ r/R = 2/5 …………………(i)

Now,

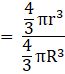
= (r/R)³

= (2/5)³ …………………[Using (i)]

= 8/125

= 8 : 125

So,

The ratio of the volumes of the given spheres will be 8 : 125