A solid is made up of a cube and a hemisphere attached on its top as shown in the figure. Each edge of the cube measures 5 cm and the hemisphere has a diameter of 4.2 cm. Find the total area to be painted.
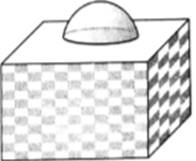
Total area to be painted = TSA of cube + CSA of hemisphere – Base area of hemisphere
[TSA = Total surface area & CSA = Curved surface area]
Given,
Diameter of hemisphere = 4.2 cm
Radius of hemisphere, r = 2.1 cm ![]()
Side of cube, a = 5 cm
And
TSA of cube = 6a2, where a is the side of cube
CSA of hemisphere = 3πr2, where r is the base radius
Base area = πr2 [As base is circular]
Therefore,
Total area to be painted = 6a2 + 3πr2 – πr2 = 6a2+ 2πr2
![]()
= 6(25) + (2 × 22 × 0.3 × 2.1)
= 177.72 cm2
31