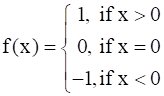
Show that the Signum Function f : R → R, given by is neither one-one nor onto.

Question

Philoid · Accepted Answer

It is given that f : R → R, given by

We can observed that f(1) = f(2) = but 1 ≠ 2.

Thus, f is not one – one.

Now, as f(x) takes only 3 values (1, 0, -1) for the element -2 in co-domain R, there exists any x in domain R such that f(x) = -2

Thus, f is not onto.

Therefore, the signum function is neither one-one nor onto.

Show that the Signum Function f : R → R, given byis neither one-one nor onto.