Find the values of k so that the function f is continuous at the indicated point in Exercises 26 to 29.

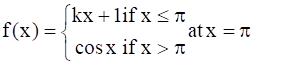
It is given that

Also, it is given that function f is continuous at x = k,

So, if f is defined at x = p and if the value of the f at x = k equals the limit of f at x = k.

We can see that f is defined at x = p and

f(π) = kπ + 1

⇒

⇒ kπ + 1 = cosπ = kπ + 1

⇒ kπ + 1 = -1 = kπ + 1

⇒ k =

Therefore, the required value of k is.