If 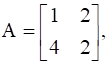 then show that |2A| = 4|A|.
then show that |2A| = 4|A|.
|A| = 
We know that determinant of A is calculated as 
= 1(2) - 2(4)
= 2 - 8
|A| = -6
LHS: |2A|


= 2(4) - 4(8)
= 8 - 32 = -24
|2A| = -24 …LHS
RHS: 4|A|
4|A|= 4(-6)
= -24
4|A| = -24 …RHS
LHS = RHS
Hence proved.
3
If 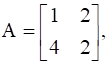 then show that |2A| = 4|A|.
then show that |2A| = 4|A|.
|A| = 
We know that determinant of A is calculated as 
= 1(2) - 2(4)
= 2 - 8
|A| = -6
LHS: |2A|


= 2(4) - 4(8)
= 8 - 32 = -24
|2A| = -24 …LHS
RHS: 4|A|
4|A|= 4(-6)
= -24
4|A| = -24 …RHS
LHS = RHS
Hence proved.