If for , – 1

Question

If for , – 1 < x < 1, prove that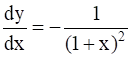

Philoid · Accepted Answer

Given,

Now, squaring both sides, we get

⇒

⇒ x² + x²y = y² + y²x

⇒ x² – y² = xy² – x²y

⇒ (x + y)(x – y) = xy (y – x)

⇒ x + y = –xy

⇒ y + xy = –x

⇒ y (1 + x) = –x

⇒

Differentiating both sides with respect to x, we get

Using Quotient Rule

∴

Hence, Proved