Prove that the determinant 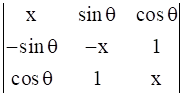is independent of θ.

Let Δ =

Expanding the above determinant along R₁ i.e. Row 1

Δ = x (-x² – 1) – sinθ (-x × sinθ – cosθ × 1) + cosθ (-sinθ × 1 + x cosθ)

Δ = -x³ – x + xsin²θ + sinθ × cos θ – sinθ × cosθ + x cos²θ

Δ = -x³ – x + x (sin²θ + cos²θ)

Since, sin²θ + cos²θ = 1

∴ Δ = -x³ – x + x

Hence Δ is independent of θ.