Prove that 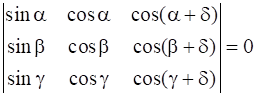
Let Δ = 
Δ = 
Δ = 
Applying Elementary Column Transformations
C1→ C1 + C3
Δ = 
Since, the two columns are identical
[In a determinant if two columns are identical the the value of determinant is 0]
So, the value of given determinant is 0
∴ Δ = 0
Hence, the given result is proved.
15