Find the values of x, y, z if the matrix satisfy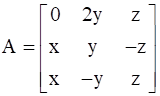 
the equation A’A =I.
Given A 
Transpose of a matrix: If A be an m×n matrix, then the matrix obtained by interchanging the rows and columns of A is called the transpose of A. It is denoted by A’ or AT.
∴ Transpose of A = A’ =
Given equation
A’A =I
=
=
. =
As these matrices are equal to each other that means each element of matrix on L.H.S is equal each element of matrix on R.H.S.
∴ On comparing elements on both sides we get
4y2 + z2 = 1 …… (1)
2y2 – z2 = 0 …… (2)
x2 + y2 + z2 = 1 …… (3)
– y2 – z2 = 0 …… (4)
From equation (4) we get,
x2 = y2 + z2 …… (5)
Substituting this value in equation (3) we get,
2y2 + 2z2 = 1 …… (6)
Subtracting equation (2) and (6) we get,
3z2 = 1
z2 = 1/3
z = 
1/3
Substituting value of z in equation (2) we get,
2y2 = 1/3
y2 = 1/6
y = �1/6
Substituting values of y and z in equation (5) we get,
x2 = 1/2
x = �1/2
Hence values of x, y, z are �1/2, �1/6, �1/3 respectively.