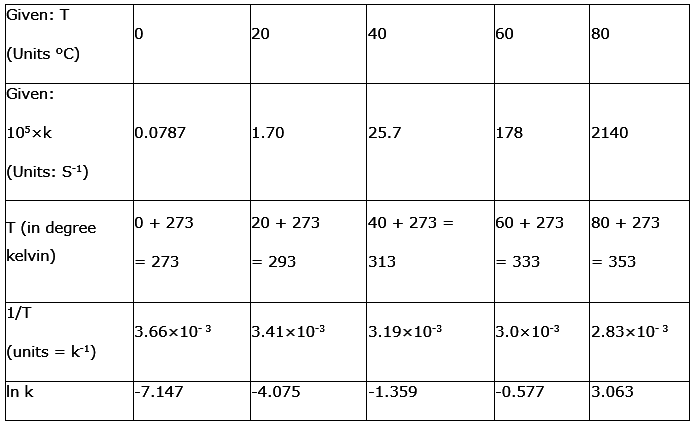
The rate constant for the decomposition of N₂O₅ at various temperatures is given below:

T/°C	0	20	40	60	80
105 × k/s-1	0.0787	1.70	25.7	178	2140

Draw a graph between ln k and 1/T and calculate the values of A and Ea. Predict the rate constant at 30° and 50°C.

To convert the temperature in °C to °K we add 273 K.

The graph is given as:

The Arrhenius equation is given by k = Ae^-Ea/RT

Where, k- Rate constant

A- Constant

E_a-Activation Energy

R- Gas constant

T-Temperature

Taking natural log on both sides,

ln k = ln A-(E_a/RT)

→ equation 1

By plotting a graph, ln K Vs 1/T, we get y-intercept as ln A and Slope is –E_a/R.

Slope = (y₂-y₁)/(x₂-x₁)

By substituting the values, slope = -12.301

⇒ –E_a/R = -12.301

But, R = 8.314 JK^-1mol^-1

⇒ E_a = 8.314 JK^-1mol^-1 × 12.301 K

⇒ E_a = 102.27 kJ mol^-1

Substituting the values in equation 1 for data at T = 273K

⇒

⇒

(∵ At T = 273K, ln k = -7.147)

On solving, we get ln A = 37.911

∴ A = 2.91×10⁶

When T = 30⁰C, hence T = 30 + 273 = 303K

⇒

⇒

⇒ ln k = -2.8

⇒ k = 6.08x10^-2s^-1.

When T = 50⁰C, hence T = 50 + 273 = 323K

Substituting in equation 1,

 A = 2.91 × 10⁶, E_a = 102.27 kJ mol^-1

Ln K = ln(2.91 × 10⁶)- 102.27/(8.314 × 323)

Thus, ln k = -0.5 and k = 0.607s^-1.