Solve each of the following quadratic equations:

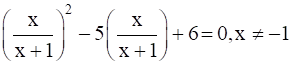
Given: - - - - - - - - (1)

Let

y² - 5y + 6 = 0 substituting value for y in (1)

Using the splitting middle term - the middle term of the general equation is divided in two such values that:

Product = a.c

For the given equation a = 1 b = - 5 c = 6

= 1.6 = 6

And either of their sum or difference = b

= - 5

Thus the two terms are - 3 and - 2

Difference = - 3 - 2 = - 5

Product = - 3. - 2 = 6

y² - 5y + 6 = 0

y² - 3y - 2y + 6 = 0

y(y - 3) - 2 (y - 3) = 0

(y - 3)(y - 2) = 0

(y - 3) = 0 or (y - 2) = 0

y = 3 or y = 2

Case I: if y = 3

x = 3x + 3

2x + 3 = 0

x = - 3/2

Case II: if y = 2

x = 2x + 2

x = - 2

Hence the roots of equation are