Integrate the functions.
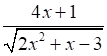
Let 4x + 1 = ![]()
⇒ 4x + 1 = A(4x + 1) + B
⇒ 4x + 1 = 4Ax+ A+ B
Now, equating the coefficients of x and constant term on both sides, we get,
4A = 4
⇒ A = 1
A + B = 1
⇒ B = 0
Let 2x2 + x – 3 =t
⇒ (4x + 1) dx = dt
⇒ 
⇒ ![]()
![]() 2
2![]()
16
Integrate the functions.
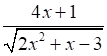
Let 4x + 1 = ![]()
⇒ 4x + 1 = A(4x + 1) + B
⇒ 4x + 1 = 4Ax+ A+ B
Now, equating the coefficients of x and constant term on both sides, we get,
4A = 4
⇒ A = 1
A + B = 1
⇒ B = 0
Let 2x2 + x – 3 =t
⇒ (4x + 1) dx = dt
⇒ 
⇒ ![]()
![]() 2
2![]()