Integrate the rational functions.

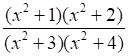
Now,

Let

4x² + 10 = (Ax + B)(x² + 4) + (Cx + D)(x² + 3)

⇒ 4x² + 10 = Ax³ + 4Ax + Bx² + 4B + Cx³ + 3Cx + Dx² + 3D

⇒ 4x² + 10 = (A+C)x³ + (B + D)x² + (4A + 3C)x + (4B + 3D)

Equating the coefficients of x³, x², x and constant term, we get,

A + C = 0

B + D = 0

4A + 3C = 0

4B + 3 B = 0

On solving these equations, we get,

A = 0, B = -2, C = 0 and D = 6

Therefore,