Show that the three lines with direction cosines 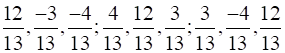are mutually perpendicular.

We know that

If l₁, m₁, n₁ and l₂, m₂, n₂ are the direction cosines of two lines; and θ is the acute angle between the two lines; then cos θ = |l₁l₂ + m₁m₂ + n₁n₂|

If two lines are perpendicular, then the angle between the two is θ = 90°

⇒ For perpendicular lines, | l₁l₂ + m₁m₂ + n₁n₂ | = cos 90° = 0, i.e.

| l₁l₂ + m₁m₂ + n₁n₂ | = 0

So, in order to check if the three lines are mutually perpendicular, we compute | l₁l₂ + m₁m₂ + n₁n₂ | for all the pairs of the three lines.

Now let the direction cosines of L₁, L₂ and L₃ be l₁, m₁, n₁; l₂, m₂, n₂ and l₃, m₃, n₃.

First, consider

⇒

⇒

⇒ L₁⊥ L₂ ……(i)

Next, consider

⇒

⇒

⇒ L₂⊥ L₃ …(ii)

Now, consider

⇒

⇒

⇒ L₁⊥ L₃ …(iii)

∴ By (i), (ii) and (iii), we have

L₁, L₂ and L₃ are mutually perpendicular.