A homogeneous differential equation of the from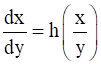 
can be solved by making the substitution.
(A) (4x + 6y + 5)dy – (3y + 2x + 4)dx = 0
It cannot be homogeneous as we can see that (4x + 6y + 5) is not homogeneous.
(B) (xy)dx – (x3 + y3)dy = 0
It cannot be homogeneous as the xy which multiplies with dx and x3 + y3 which multiplies with dy are not of same degree.
(C) (x3 + 2y2)dx + 2xy dy = 0
Similarly, it cannot be homogeneous as the x3 + 2y2 which multiplies with dx and 2xy which multiplies with dy are not of same degree.
(D) y2dx + (x2 – xy – y2)dy = 0
Here, putting x = kx and y = ky
= k0.f(x,y)
Therefore, the given differential equations is homogeneous.
17