For each of the differential equations given in question, find the general solution:

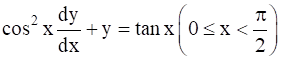
It is given that

This is equation in the form of (where, p = and Q =)

Now, I.F. =

Thus, the solution of the given differential equation is given by the relation:

y(I.F.) =

-----------(1)

Now, Let t = tanx

⇒ sec²xdx = dt

Thus, the equation (1) becomes,

⇒ te^tanx = (t – 1)e^t + C

⇒ te^tanx = (tanx – 1)e^tanx + C

⇒ y = (tanx -1) + C e^-tanx

Therefore, the required general solution of the given differential equation is

y = (tanx -1) + C e^-tanx.