For each of the exercises given below, verify that the given function (implicit or explicit) is a solution of the corresponding differential equation.
x2 = 2y2 log y :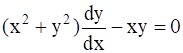 
It is given that x2 = 2y2 log y
Now, differentiating both sides w.r.t. x, we get,
2x = 2.
Now, substituting the value of 
in the LHS of the given differential equation, we get,
= xy –xy
= 0
Therefore, the given function is the solution of the corresponding differential equation.
2