A stone of mass m tied to the end of a string revolves in a vertical circle of radius R.
The net forces at the lowest and highest points of the circle directed vertically downwards are: [Choose the correct alternative]
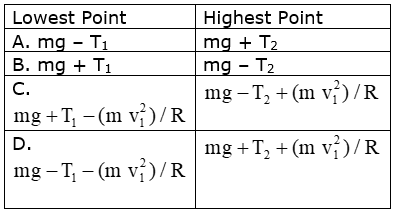
T1 and v1 denote the tension and speed at the lowest point. T2 and v2 denote corresponding values at the highest point.
Given:
Mass of body = m Kg
Radius of vertical circle = R m
From the free body diagram of body at lowest point, we can write,

According Newton’s 2nd law of motion,
Fnet = mg-T
Fnet = mv12/R (Centrifugal force)
mg-T = mv12/R …(1)
Where,
v1 = Velocity at lowest point.
m = mass of body
R = radius of path
T = tension in string
From the free body diagram of body at highest point , we can write,

Fnet = T + mg
Fnet = mv22/ R
Where,
v2 = velocity of body at highest point,
T + mg = mv22/ R …(2)
From equation (1) and (2) we conclude that option (1) is correct.