Two identical ball bearings in contact with each other and resting on a frictionless table are hit head-on by another ball bearing of the same mass moving initially with a speed V. If the collision is elastic, which of the following (Fig. 6.14) is a possible result after collision?
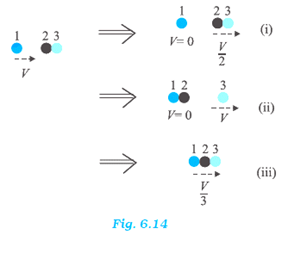
From the figure, it is observed that the linear momentum is conserved in all the cases. For an elastic collision, kinetic energy is also conserved.
Let us assume that the mass of each ball is m.
Total kinetic energy before collision,
Ki = (1/2)mV2 + (1/2)m(0)2 + (1/2)m(0)2
⇒ Ki = (1/2)mV2
Case (i):
Total kinetic energy after collision,
Kf = (1/2)m(0)2 + (1/2)m(V/2)2 + (1/2)m(V/2)2
⇒ Kf = (1/8)mV2 + (1/8)mV2
⇒ Kf = (1/4)mV2
The total kinetic energy of the system before collision is not equal to the total kinetic energy after collision in this case. The total kinetic energy is not conserved. Hence, the collision is not elastic.
Case (ii):
Total kinetic energy after collision,
Kf = (1/2)m(0)2 + (1/2)m(0)2 + (1/2)mV2
⇒ Kf = (1/2)mV2
The total kinetic energy of the system before collision is equal to the total kinetic energy after collision in this case. The total kinetic energy is conserved. Hence, the collision is elastic.
Case (iii):
Total kinetic energy after collision,
Kf = (1/2)m(V/3)2 + (1/2)m(V/3)2 + (1/2)m(V/3)2
⇒ Kf = (1/18)mV2 + (1/18)mV2 + (1/18)mV2
⇒ Kf = (1/6)mV2
The total kinetic energy of the system before collision is not equal to the total kinetic energy after collision in this case. The total kinetic energy is not conserved. Hence, the collision is not elastic.
Thus, only case (ii) is an elastic collision.