Consider the decay of a free neutron at rest: ![]()
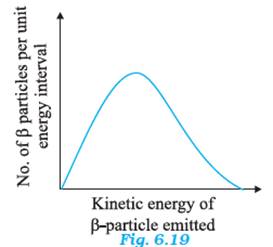
Show that the two-body decay of this type must necessarily give an electron of fixed energy and, therefore, cannot account for the observed continuous energy distribution in the β-decay of a neutron or a nucleus (Fig. 6.19).
[Note: The simple result of this exercise was one among the several arguments advanced by W. Pauli to predict the existence of a third particle in the decay products of β-decay. This particle is known as neutrino. We now know that it is a particle of intrinsic spin � (like e—, p or n), but is neutral, and either massless or having an extremely small mass (compared to the mass of electron) and which interacts very weakly with matter. The correct decay process of neutron is: ![]() ]
]
Let the masses of electron and proton be m and m’ respectively and their velocities be v and v’ respectively.
Using law of conservation of linear momentum,
Momentum of electron + momentum of proton = momentum of neutron
mv + m’v’ = 0
⇒ v’ = -mv/m’
This shows that the electrons and protons move in the opposite directions. If mass Δm has been converted into energy, then
(1/2)mv2 + (1/2)m’v’2 = Δmc2
⇒ (1/2)mv2 + (1/2)m’(-mv/m’)2 = Δmc2
⇒ (1/2)mv2(1+m/m’) = Δmc2
⇒ 
⇒ 
All the quantities on the RHS of the above equation are constant. Hence, v2 is also constant. It shows that the emitted electron must have a constant energy. Hence, we cannot account for the observed continuous energy distribution in the β-decay of a neutron or a nucleus.