Use the formula 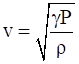to explain why the speed of sound in air

(a) is independent of pressure,

(b) increases with temperature,

(c) increases with humidity.

(a) Given:

…(1)

Where,

γ = adiabatic coefficient / index

P = pressure

ρ = Density of medium

ρ = Mass (M) /Volume (V)

By putting the value of density, in equation (1), it can be reduced to,

…(2)

Ideal gas equation is written as,

PV = RT …(3) (for n = 1)

Since, R is a constant, For constant Temperature, PV is constant.

From equation (2), we see that γ and M are also constant,

So it can be concluded that, velocity of sound is constant at constant temperature, it is not affected by change in pressure.

(b)

Ideal gas equation is written as,

PV = RT (for n = 1)

P = RT/V

The equation of velocity can be rewritten as,

…(5)

We know that V×ρ (i.e. mass), γ and R are constants.

We can write the equation (5) as,

Hence the speed of sound in a medium is proportional to the square root of the temperature of the gaseous medium.

(c) Let v_m, ρ_m and v_d, ρ_d be the speed of the sound and density in the moist air and dry air respectively.

From the equation,

The velocity in moist air and dry air can be written as

…(6)

…(7)

By dividing equation (6) by equation (7), we get,

…(8)

The presence of moisture in air reduces the density of air,

i.e. ρ_d> ρ_m

so, v_m>v_d.

Hence, the speed of sound in moist air is greater than speed in dry air. Thus in gaseous medium, the speed of sound increases with humidity.