The transverse displacement of a string (clamped at its both ends) is given by

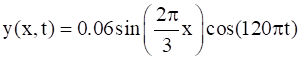
Where x and y are in m and t in s. The length of the string is 1.5 m and its mass is 3.0 ×10^–2 kg.

Answer the following:

(a) Does the function represent a travelling wave or a stationary wave?

(b) Interpret the wave as a superposition of two waves travelling in opposite directions. What is the wavelength, frequency, and speed of each wave?

(c) Determine the tension in the string.

Given:

…(1)

(a) The general equation of an standing wave is given by,

y(x, t) = 2 sin kx × cos wt …(2)

The equation 1, is similar to equation 2, hence the given equation represents a standing wave.

(b) A wave travelling along the positive x-direction is given by,

y₁ = a sin(wt-kx)

For a wave travelling in negative x direction is given by,

y₂ = a sin (wt + kx)

The superposition of these two waves can be written as,

y = y₁ + y₂

y = a sin (wt-kx) – a sin(wt + kx)

The terms can be expanded using the formula of sin(a + b) and the result is,

y = 2 a sin(kx) cos(wt)

y = 2a sin (2πx/λ) cos(2πvt) …(3)

By comparing equation (1) and (3) we have,

2 π/λ = 2 π/3

⇒ wavelength, λ = 3 m

It is given that,

120 π = 2 πv

Frequency, v = 60Hz

Wave speed, V is given by,

V = v× λ

V = 60 s^-1× 3m

V = 180 ms^-1

(c) The velocity of a transverse wave in a string is given by,

V = (T/m)^1/2

Where,

V = velocity of wave,

T = tension in string

m = mass per unit length of string

⇒ m = mass of the string / length of the string

⇒ m = 3.02× 10^-2Kg/ 1.5 m

⇒ m = 2× 10^-2 Kgm^-1

Tension in the string, T = V²m

T = 2× 10^-2Kgm^-1× (180ms^-1)²

T = 648 N