If a, b, c are in A.P.; b, c, d are in G.P. and

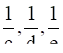are in A.P. prove that a, c, e are in G.P.

It is given that a, b, c are in AP

∴ b = (a + c)/2 …(1)

Also given that b, c, d are in GP

∴ c² = bd …(2)

Also,

are in AP

So, their common difference is same

…(3)

We need to show that a, c, e are in GP

i.e c² = ae

From (2), we have

c² = bd

Putting value of

⇒ c(c + e) = e(a + c)

⇒ c² + ce = ea + ec

⇒ c² = ea

Thus, a, c, e are in GP.

Hence, Proved.