Find 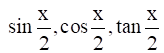in the following:

tan x = –4/3, x in quadrant II

Given that x is in quadrant II

So,

90° < x < 180°

Dividing with 2 all sides

(90°/2) < x/2 < (180°/2)

45° < x/2 < 90°

∴ lies in 1^st quadrant

In 1^st quadrant,

sin, cos & tan are positive

⇒ are positive

Given

tan x = -(4/3)

We know that

Replacing x with x/2

Replacing by a

⇒ 4a²-6a-4 = 0

⇒ 4a²-8a+2a-4 = 0

⇒ 4a(a-2)+2(a-2) = 0

⇒ (4a+2)(a-2) = 0

∴ a = -1/2 or a = 2

Hence,

or

∵ lies in 1^st quadrant

is positive

∴

Now,

We know that

1 + tan²x = sec²x

Replacing x with x/2

∵ lies in 1^st quadrant

is positive in 1^st quadrant

We know that-

Replacing x with x/2

Hence,