The first and last term of an A.P. are a and l respectively. If S is the sum of all the terms of the A.P. and the common difference is given by 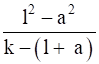 , then k=
, then k=
Let the common difference and number of terms of AP be d and n respectively.
Last term of AP = an = l (given)
l = a + (n–1) d
n = ![]() + 1……………… (1)
+ 1……………… (1)
S= ![]() (2a + (n–1) d)
(2a + (n–1) d)
S = ![]() + 1) (2a + (
+ 1) (2a + (![]() + 1 –1) d) …………… using (1)
+ 1 –1) d) …………… using (1)
S= ![]() + 1) (2a + l –a)
+ 1) (2a + l –a)
S= ![]() + 1) (a + l)
+ 1) (a + l)
(![]() + 1) =
+ 1) = ![]()
![]() =
= ![]() –1
–1
![]() =
= ![]()
D = ![]()
Comparing this with 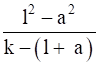
We get k =2S
10