Surface area of a cone is 188.4 sq.cm and its slant height is 10cm. Find its perpendicular height (π = 3.14)

Given: Surface area of cone (SA) = 188.4 cm²

Slant height of cone (l) = 10 cm

So, we need to write the formula of curved surface area of cone with slant height l,

Surface Area = πrl

Where, r = radius of base of cone

Substituting values in the formula, we get

188.4 = 3.14×r×10

⇒ 188.4 = 31.4r

⇒ r = 188.4/31.4

⇒ r = 6

So we have,

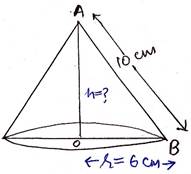
We need to find the perpendicular height, h.

Using Pythagoras theorem in ∆AOB, we get

AB² = AO² + OB²

⇒ AO² = AB² – OB²

⇒ h² = 10² – 6²

⇒ h² = 100 – 36 = 64

⇒ h = √64 = 8

Thus, perpendicular height of the cone is 8 cm.