P (a, b) is the mid-point of a line segment between axes. Show that equation of the line is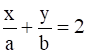

Let AB be a line segment whose midpoint is P (a, b).

Let the coordinates of A and B be (0, y) and (x, 0) respectively.

We know that the midpoint formula is given by .

Since P is the midpoint of (a, b),

⇒

⇒ a = x/2 and b = y/2

⇒ x = 2a and y = 2b

∴ A = (0, 2b) and B = (2a, 0)

We know that the equation of the line passing through the points (x₁, y₁) and (x₂, y₂) is given by

∴

⇒

⇒

⇒ a (y – 2b) = -bx

⇒ ay – 2ab = -bx

⇒ bx + ay = 2ab

Dividing by ab on both sides,

⇒

⇒

Ans. The equation of line is .