In Fig. 8.7, P is the mid-point of side BC of a parallelogram ABCD such that ∠BAP = ∠DAP. Prove that AD = 2CD.
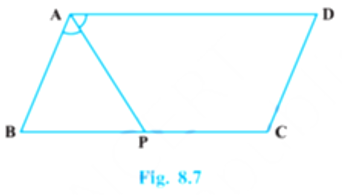
Since ABCD is a parallelogram, AD||BC and AB is a transversal.
![]() (Sum of co-interior angles is 180
(Sum of co-interior angles is 180![]() )
)
![]()
In ΔABP,
![]()


![]()
![]() (opposite sides of equal angles are equal)
(opposite sides of equal angles are equal)
Now, multiply both sides by 2, we get,
![]()
![]() (P is mid-point of BC)
(P is mid-point of BC)
![]() (AB||CD and AD||BC)
(AB||CD and AD||BC)
Hence, Proved.
10