In Fig. 10.3, if OA = 5 cm, AB = 8 cm and OD is perpendicular to AB, then CD is equal to:
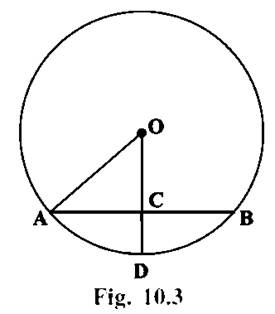
Given:
Radius of the circle = r = AO = 5 cm
Length of chord AB = 8 cm
Since the line drawn through the center of a circle to bisect a chord is perpendicular to the chord, therefore AOC is a right angled triangle with C as the bisector of AB.
∴ AC = 1/2(AB) = 8/2 = 4 cm
In right angled triangle AOC, by Pythagoras theorem, we have:
(AO)2 = (OC)2 + (AC)2
⇒ (5)2 = (OC)2 + (4)2
⇒ (OC)2 = (5)2 - (4)2
⇒ (OC)2 = 25 – 16
⇒ (OC)2 = 9
Take square root on both sides:
⇒ (OC) = 3
∴ The distance of AC from the center of the circle is 3 cm.
Now, OD is the radius of the circle, ∴ OD = 5 cm
CD = OD – OC
CD = 5 – 3
CD = 2
∴ CD = 2 cm
2