In Fig. 10.10, if AOB is a diameter and ADC = 120°, then CAB = 30°

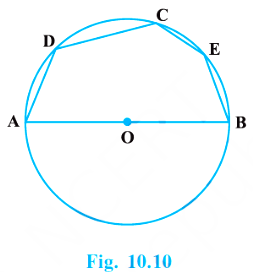
Let AOB be the diameter of the circle.

Given: ∠ADC = 120°

Firstly, join CB.

Then, we have a cyclic quadrilateral ABCD.

Since sum of opposite angles of cyclic quadrilateral is 180°, therefore

∠ADC + ∠ABC = 180°

⇒ 120° + ∠ABC = 180°

⇒ ∠ABC = 180° - 120°

⇒ ∠ABC = 60°

Now join AC.

Also, diameter subtends a right angle to the circle,

∴ In ΔABC, ∠ACB = 90°

Now, by angle sum property of a triangle, sum of all angles of a triangle is 180°.

∴ ∠CAB + ∠ABC + ∠ACB = 180°

⇒ ∠CAB + 60° + 90° = 180°

⇒ ∠CAB = 180° - 90° - 60°

⇒ ∠CAB = 30°

∴ The given statement is true.