In Fig.10.13, ∠ ADC = 130° and chord BC = chord BE. Find ∠ CBE.

Question

Philoid · Accepted Answer

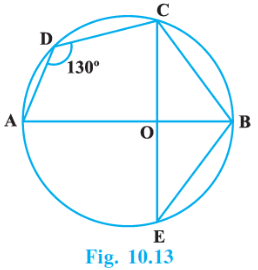
Given: ∠ADC = 130°

chord BC = chord BE

Since, ABCD is a cyclic quadrilateral, therefore sum of its opposite angles is 180°.

⇒ ∠ADC + ∠OBC = 180°

⇒ 130° + ∠OBC = 180°

⇒ ∠OBC = 50°

Now, in triangles BOC and BOE:

OB = OB (common)

BC = BE (given)

OC = OE (radius of the circle)

By SSS congruence rule, ΔBOC ≅ ΔBOE.

∴ ∠OBC = ∠OBE = 50° (By CPCT)

Now, ∠CBE = ∠CBO + ∠OBE

∠CBE = 50° + 50°

∠CBE = 100°

In Fig.10.13, ∠ADC = 130° and chord BC = chord BE. Find ∠CBE.