The dimensions of a rectangle ABCD are 51 cm × 25 cm. A trapezium PQCD with its parallel sides QC and PD in the ratio 9:8, is cut off from the rectangle as shown in the Fig. 12.6. If the area of the trapezium PQCD is th part of the area of the rectangle, find the lengths QC and PD.

Question

Philoid · Accepted Answer

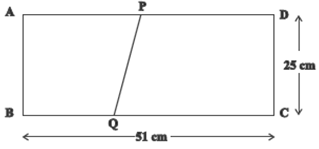
Area of rectangle (ABCD) = BC × CD

⇒ Area of rectangle (ABCD) = 51 × 25 = 1275 cm²

Area of trapezium PQCD = 5/6 × Area of rectangle (ABCD)

⇒ Area of trapezium PQCD = 5/6 × 1275 = 1062.5 cm²

Given that QC:PD = 9:8

Let QC = 9x and PD = 8x

Area (PQCD) =

⇒ Area (PQCD) =

⇒ 1062.5 =

⇒ 85 = 17x

⇒ x = 5cm

QC = 9x = 45cm

PD = 8x = 40 cm