In Fig. 6.1, if AB || CD || EF, PQ || RS, ∠RQD = 25° and ∠CQP = 60°, then ∠QRS is equal to

Question

In Fig. 6.1, if AB || CD || EF, PQ || RS, ∠ RQD = 25° and ∠ CQP = 60°, then ∠ QRS is equal to

Philoid · Accepted Answer

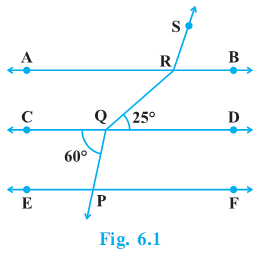
From the given figure, we have

AB || CD || EF

PQ || RS

∠RQD = 25°

∠CQP = 60°

Now, we have PQ || RS.

We know if a transversal intersects two parallel lines, then each pair of alternate exterior angles is equal.

Since, PQ || RS

⇒ ∠PQC = ∠BRS

We have ∠PQC = 60°

⇒ ∠BRS = 60° - - - - (i)

We know, if a transversal intersects two parallel lines, then each pair of alternate interior angles is equal.

Since, AB || CD

⇒ ∠DQR = ∠QRA

We have ∠DQR = 25°

⇒ ∠QRA = 25° - - - - (ii)

By linear pair axiom,

∠ARS + ∠BRS = 180°

⇒ ∠ARS = 180° - ∠BRS

⇒ ∠ARS = 180° - 60° (From (i), ∠BRS = 60°)

⇒ ∠ARS = 120° - - - - (iii)

Now, ∠QRS = ∠QRA + ∠ARS

From equations (ii) and (iii), we have ∠QRA = 25° and ∠ARS = 120°

Thus, the above equation can be written as:

∠QRS = 25° + 120°

⇒ ∠QRS = 145°

Thus, the option (C) is correct.

Option (A) is not correct because 85°, i.e., 60° + 25° is not the correct angle combination for ∠QRS.

Option (B) is not correct because 135°, is not the exact solution of ∠QRS.

Option (D) is not correct because 110°, is not the correct solution of ∠QRS.