In Fig. 6.3, if OP||RS, ∠OPQ = 110° and ∠QRS = 130°, then ∠PQR is equal to

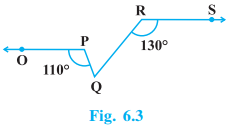
In the figure, it is given to us that –

OP || RS

∠OPQ = 110°

∠QRS = 130°

We have to find ∠PQR.

Let us extend OP, so as to intersect QR at point D. The figure is as -

Now, we have OP || RS and, DR is a transversal.

We know, if a transversal intersects two parallel lines, then each pair of alternate angles are equal.

⇒ ∠DRS = ∠RDP

It is given to us that ∠QRS = 130°

⇒ ∠DRS = 130°

⇒ ∠RDP = 130° - - - - (i)

Now, we have QR as a line segment. By linear pair axiom,

∠PDQ + ∠PDR = 180°

⇒ ∠QDP + ∠RDP = 180°

⇒ ∠QDP = 180° - ∠RDP

⇒ ∠QDP = 180° - 130°

⇒ ∠QDP = 50°

⇒ ∠PDQ = 50° - - - - (ii)

In ΔPQD,

∠OPQ is an exterior angle.

Also, we know that exterior angle is equal to the sum of the two interior opposite angles.

⇒ ∠OPQ = ∠PQD + ∠PDQ - - - - (iii)

We have ∠OPQ = 110° and from equation (ii) we have ∠PDQ = 50°.

So, equation (iii) can be written as –

110° = ∠PQD + 50°

⇒ ∠PQD = 60°

So, ∠PQR = 60°

Thus, option (C) is correct.

Option (A) is not correct. If ∠PQR is equal to 40°, then it won’t satisfy the linear axiom. So, ∠PQR is not 40°.

Option (B) is not correct. We have got ∠PDQ = 50°. If ∠PQR = 50°, then the exterior angle, i.e., ∠OPQ = 110° is not equal to the sum of the opposite interior angles, ∠PDQ and ∠PQR which are now 50° each. So, ∠PQR is not equal to 50°.

Option (D) is not correct. We have, ∠OPQ = 110°. By linear pair axiom, ∠QPD = 70°. So, ∠PQR is not equal to 70⁰