In Fig. 6.13, BA || ED and BC || EF. Show that ∠ABC + ∠DEF = 180°

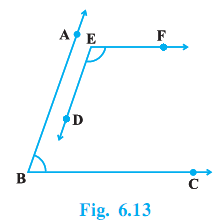
It is given to us –

BA || ED
BC || EF

To show that - ∠ABC + ∠DEF = 180°

Let us extend DE to intersect BC at G, and EF to intersect BA at H. Then, the figure becomes –

Since, BA || DE

⇒ BA || GE

We have two parallel lines BA and GE, and BG is a transversal intersecting BA and GE at points B and G respectively.

⇒ ∠ABC = ∠EGC - - - - (i)

Also, BC || EF, and GE is a transversal intersecting BC and EF at points G and E respectively.

⇒ ∠EGC = ∠HEG - - - - (ii)

Since GE is a ray standing on the line HF. By linear pair axiom,

∠HEG + ∠GEF = 180°

⇒ ∠EGC + ∠GEF = 180° [From equation (ii)]

⇒ ∠ABC + ∠GEF = 180°

⇒ ∠ABC + ∠DEF = 180°

Hence, proved.