In Fig. 6.14, DE || QR and AP and BP are bisectors of ∠EAB and ∠RBA, respectively. Find ∠APB.

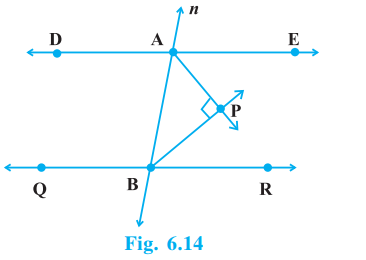
It is given to us –

DE || QR and n is the transversal intersecting DE and QR at points A and B respectively.

We have to find ∠APB.

AP and BP are the bisectors of ∠EAB and ∠ABR respectively.

⇒ ∠EAP = ∠PAB

⇒ ∠EAB = 2 × ∠PAB - - - - (i)

Also, ∠RBP = ∠PBA

⇒ ∠RBA = 2 × ∠PBA - - - - (ii)

Since, DE || QR,

∠RBA = ∠EAn (Corresponding angles)

⇒ ∠RBA = 180° - ∠EAB (By linear pair axiom, ∠EAn + ∠EAB = 180°)

Using equations (i) and (ii) in the above equation,

2 × ∠PBA = 180° - (2 × ∠PAB)

⇒ ∠PBA = 90° - ∠PAB (Dividing both sides by 2)

⇒ ∠PBA + ∠PAB = 90° - - - - (iii)

Now, in ΔPAB,

∠PBA + ∠PAB + ∠APB = 180° (Sum of three angles of a triangle is 180°)

⇒ 90° + ∠APB = 180° [From equation (iii)]

⇒ ∠APB = 90°

Thus, the value of ∠APB is 90°.