Bisectors of interior ∠B and exterior ∠ACD of a ΔABC intersect at the point T. Prove that ∠BT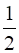C = ∠BAC.

Let us draw the figure as below –

Here, we have

A ΔABC

BC is extended to D.

Let BT be the bisector of ∠B of the triangle.

Also, let us assume the bisector of ∠ACD to be CT.

It is given that BO and CT intersect at point T.

We have to prove that ∠BTC = 1/2∠BAC

We know, if a side of a triangle is produced, the exterior angle so formed is equal to the sum of the two interior opposite angles. Here, ∠ACD is an exterior angle and the two interior opposite angles are ∠ABC and ∠CAB.

⇒ ∠ACD = ∠ABC + ∠CAB

Dividing both sides of the equation by 2,

1/2∠ACD = 1/2∠ABC + 1/2∠CAB

⇒ ∠TCD = 1/2∠ABC + 1/2∠CAB (∠TCD = 1/2∠ACD, since CT is the bisector of ∠ACD) - - - - (i)

Again, in ΔBTC, ∠TCD is an exterior angle of the triangle and the two opposite interior angles are ∠BTC and ∠CBT.

⇒∠TCD = ∠BTC + ∠CBT

⇒ ∠TCD = ∠BTC + 1/2∠ABC (∠CBT = 1/2∠ABC, since BT is the bisector of ∠ ABC) - - - - (ii)

From equations (i) and (ii), we can say that

1/2∠ABC + 1/2∠CAB = ∠BTC + 1/2∠ABC

⇒ 1/2∠CAB = ∠BTC

⇒ ∠BTC = 1/2 ∠CAB

⇒ ∠BTC = 1/2 ∠BAC

Hence, proved.