In Fig. 6.17, ∠ Q ∠ R, PA is the bisector of ∠ QPR and PM ⊥ QR. Prove that ∠ APM = ( ∠ Q – ∠ R).

Question

In Fig. 6.17, ∠ Q > ∠ R, PA is the bisector of ∠ QPR and PM ⊥ QR. Prove that ∠ APM = ( ∠ Q – ∠ R).

Philoid · Accepted Answer

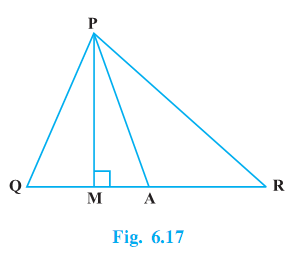
It is given to us –

∠Q > ∠R

PA is the bisector of ∠QPR

⇒ ∠QPA = ∠RPA - - - - (i)

PM ⊥ QR

⇒ ∠PMR = ∠PMQ = 90° - - - - (ii)

We have to prove that ∠APM = 1/2 (∠Q - ∠R)

Since, the sum of the three angles of a triangle is equal to 180°, in ΔPQM,

∠PQM + ∠PMQ + ∠QPM = 180°

⇒ ∠PQM + 90° + ∠QPM = 180° [From equation (ii)]

⇒ ∠PQM + ∠QPM = 180° - 90°

⇒ ∠PQM + ∠QPM = 90°

⇒ ∠PQM = 90° - ∠QPM - - - - (iii)

Similarly, in ΔPMR, the sum of the three angles of a triangle is equal to 180°

⇒ ∠PMR + ∠PRM + ∠RPM = 180°

⇒ 90° + ∠PRM + ∠RPM = 180° [From equation (ii)]

⇒ ∠PRM + ∠RPM = 180° - 90°

⇒ ∠PRM + ∠RPM = 90°

⇒ ∠PRM = 90° - ∠RPM - - - - (iv)

Now, on subtracting equation (iv) from equation (iii), we get

∠PQM - ∠PRM = (90° - ∠QPM) - (90° - ∠RPM) ×

⇒ ∠Q - ∠R = 90° - ∠QPM - 90° + ∠RPM

⇒ ∠Q - ∠R = ∠RPM - ∠QPM

⇒ ∠Q - ∠R = (∠RPA + ∠APM) - (∠QPA - ∠APM)

⇒ ∠Q - ∠R = ∠RPA + ∠APM - ∠QPA + ∠APM

⇒ ∠Q - ∠R = ∠QPA + 2 × ∠APM - ∠QPA [From equation (i)]

⇒ ∠Q - ∠R = 2 × ∠APM

⇒ ∠APM = 1/2 (∠Q - ∠R)

Hence, proved.

In Fig. 6.17, ∠Q > ∠R, PA is the bisector of ∠QPR and PM ⊥ QR. Prove that ∠APM = (∠Q – ∠R).