For each diagonal of the quadrilateral shown, check whether the other two corners are inside, on or outside the circle with that diagonal as diameter.

Now we have been given a quadrilateral ABCD:
Case 1 : let us now draw a diagonal BD in quadrilateral ABCD
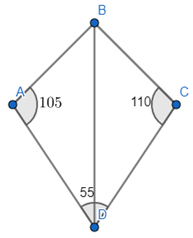
● Draw a circle considering, BD as a diagonal.

● As ∠A and ∠C are larger then 90° . Thus, point A and C lies inside the circle.
(As seen above, point on a circle, such an angle is a right angle.
Point outside the circle , such an angle is larger than 90°
Point inside the circle, such an angle is smaller than 90° )
Case 2: let us now draw a diagonal AC in quadrilateral ABCD
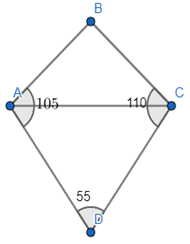
Also, By angle sum property of quadrilateral
⇒ ∠A + ∠B + ∠C + ∠D = 360°
⇒ 105° + ∠B + 110° + 55° = 360°
⇒ ∠B + 270° = 360°
⇒ ∠B = 360° – 270° = 90°
● Draw a circle considering, AC as a diagonal.
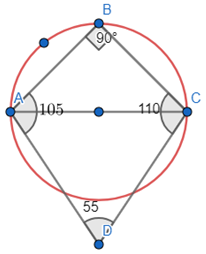
● As ∠B is 90° . Thus, point B lies on the circle.
As ∠D is smaller then 90°. Thus, point D lies outside the circle.
(As seen above, point on a circle, such an angle is a right angle.
Point outside the circle , such an angle is larger than 90°
Point inside the circle, such an angle is smaller than 90° )