What conclusion can be drawn from each part of Fig. 2.41, if
(a) DB is the bisector of ∠ADC?
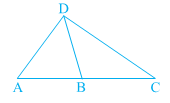
(b) BD bisects ∠ABC?
(c) DC is the bisector of ∠ADB, CA ⊥ DA and CB ⊥ DB?

a) Since, DB is the bisector of ∠ ADC.
This means that DB divides ∠ ADC into 2 equal parts.
∴ ∠ ADB = ∠ BDC
b) Since, BD bisects ∠ ABC.
This means that, ∠ ABD = ∠ DBC.
c) Since, DC is the bisector of ∠ ADB
∴ ∠ ADC = ∠ CDB ………….. (1)
Also, it is given that,
∠ CAD = ∠ CBD = 90° ……….. (2)
Also, we know that sum of interior angles of a triangle is equal to 180°.
∴ In Δ ACD,
∠ ACD+∠ CDA+ ∠ DAC =180° …. (3)
In Δ BCD,
∠ BCD+∠ CDB+∠ DBC =180° …. (4)
From (1), (2), (3), (4) we get,
∠ ACD = ∠ BCD
63