Two sides AB and BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of Δ PQR (see Fig. 7.40). Show that:
(i) Δ ABM ≅Δ PQN

(ii) Δ ABC ≅Δ PQR

Question

Philoid · Accepted Answer

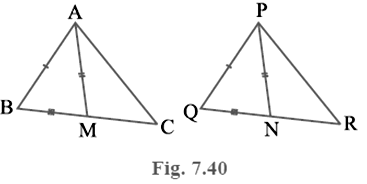
It is given in the question that:

AB = PQ

BC = QR

And,

AM = PN

(i) BC = BM

And,

QR = QN (Am and PN are medians)

BC = QR

In

AM = PN (Given)

AB = PQ (Given)

BM = QN (Proved above)

Therefore,

By SSS axiom,

(ii) In and

AB = PQ (Given)

∠ABC = ∠PQR (By c.p.c.t)

BC = QR (Given)

Therefore,

By SAS axiom,

Two sides AB and BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of Δ PQR (see Fig. 7.40). Show that:(i) Δ ABM ≅Δ PQN(ii) Δ ABC ≅Δ PQR