In Fig.9.29, ar (DRC) = ar (DPC) and ar (BDP) = ar (ARC). Show that both the quadrilaterals ABCD and DCPR are trapeziums.

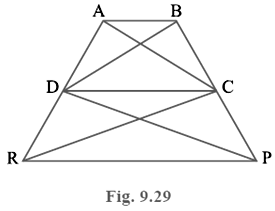
It is given that:

Area (ΔDRC) = Area (ΔDPC)

As ΔDRC and ΔDPC lie on the same base DC and have equal areas, therefore, they must lie between the same parallel lines

DC || RP

Therefore, DCPR is a trapezium. It is

Also given that:

Area (ΔBDP) = Area (ΔARC)

Area (BDP) − Area (ΔDPC) = Area (ΔARC) − Area (ΔDRC)

Area (ΔBDC) = Area (ΔADC)

Since ΔBDC and ΔADC are on the same base CD and have equal areas, they must lie between the same parallel lines

AB || CD

Therefore,

ABCD is a trapezium