A diagonal of a rectangle is inclined to one side of the rectangle at 25°. The acute angle between the diagonals is

(A) 55°

(B) 50°

(C) 40°

(D) 25°

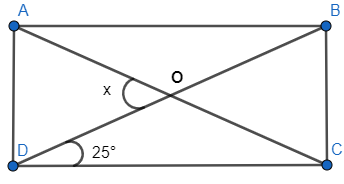
Given: ∠BDC = 25°

To Find: ∠ACD

∠ACD = ∠BCD

[ Diagonals of a rectangle are equal and thus will subtend equal angles at vertices]

∠ACD = 25°

In Δ COD,

Sum of angles of a triangle = 180°

∠ACD + ∠BCD + ∠COD = 180°

25° + 25° + ∠COD = 180°

∠COD = 180° – 50°

∠COD = 130°

∠COD + ∠AOD = 180°

[ Sum of angles on straight line = 180°]

130° + ∠AOC = 180°

∠AOC = 50°

Hence, (B) is the correct option.