The area of an equilateral triangle ABC is 17320.5cm2. With each vertex of the triangle as centre, acircle is drawn with radius equal to half the length of the side of the triangle (see Fig. 12.28). Find the area of the shaded region. (Use π = 3.14 and= 1.73205)

Question

Philoid · Accepted Answer

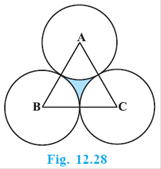
Let the side of the equilateral triangle be a

Area of equilateral triangle = 17320.5 cm²

(a)² = 17320.5

a² = 4 * 10000

a = 200 cm

Each sector is of measure 60°

Area of sector ADEF = * * r²

= * * (100)²

=

= cm²

Area of shaded region = Area of equilateral triangle - 3 × Area of each sector

= 17320.5 – 3 *

= 17320.5 – 15700

= 1620.5 cm²