Show that the four points P, Q, R, S with position vectors respectively such that are coplanar. Also, find the position vector of the point of intersection of the line segments PR and QS.

Question

Philoid · Accepted Answer

Given the position vectors of points P, Q, R and S are,, and respectively.

We have

Rearranging the terms in the above equation,

Observe that the sum of coefficients on the LHS of this equation (5 + 6 = 11) is equal to that on the RHS (2 + 9 = 11).

We now divide the equation with 11 on both sides.

Now, consider the LHS of this equation.

Let , the position vector of some point X.

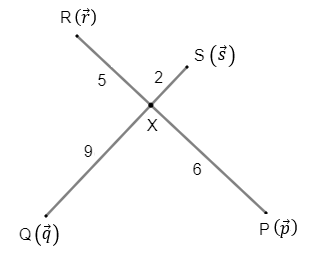
Recall the position vector of point P which divides AB, the line joining points A and B with position vectors and respectively, internally in the ratio m : n is

Here, m = 5 and n = 6

So, X divides RP internally in the ratio 5:6.

Similarly, considering the RHS of this equation, we have the same point X dividing SQ in the ratio 2:9.

So, the point X lies on both the line segments PR and QS making it the point of intersection of PR and QS.

As PR and QS are two straight lines having a common point, we have all the points P, Q, R and S lying in the same plane.

Thus, the points P, Q, R and S are coplanar and in addition, the position vector of the point of intersection of line segments PR and QS is or.