Prove by vector method that the internal bisectors of the angles of a triangle are concurrent.

Consider ΔABC with vertices A, B, C and sides BC = α, AC = β and AB = γ.

Let the position vectors of A, B and C be and respectively.

Let D and E (with position vectors and) be points on BC and AB such that AD and CE are the bisectors of ∠A and ∠C. Let, AB and CE meet at point I.

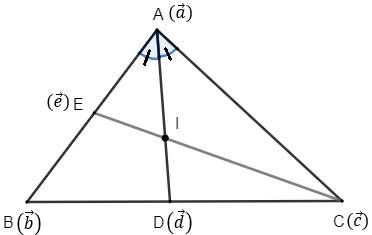
Observe from the figure that D divides BC in the ratio BD:DC.

Using the angular bisector theorem, we know that the angle bisector of an angle in a triangle bisects the opposite side in the ratio equal to the ratio of the other two sides.

(from our initial assumption)

Recall the position vector of point P which divides AB, the line joining points A and B with position vectors and respectively, internally in the ratio m : n is

Here, we have D dividing BC internally in the ratio m:n where m = γ and n = β.

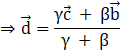
From angular bisector theorem above, we had.

Adding 1 to both sides,

In addition, as CI is the angular bisector of ∠C in ΔACD, using the angular bisector theorem, we have

So, we get

So, I divides AD in the ratio (β + γ):α.

Let the position vector of I be .

Using the aforementioned section formula, we can write

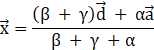
But, we already found.

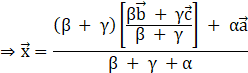
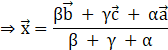
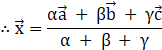
Now, observe E divides AB in the ratio AE:EB.

(from angular bisector theorem)

So, (using section formula)

By doing similar calculations as above for ∠C, we get

So, I divides CE in the ratio (α + β):γ.

Let the position vector of I now be .

Using the aforementioned section formula, we can write

But, we already found.

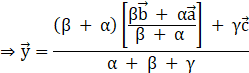
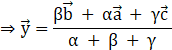
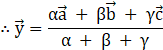
Observe that meaning the point I with position vector lies on both AB and CE.

Similarly, it can be shown that this point I also lies on the third angular bisector.

Thus, the internal bisectors of the angles of a triangle are concurrent with the point of concurrency given by the position vector where α,β and γ are sides of the ΔABC opposite to the vertices A, B and C respectively.