Find the foot of perpendicular from the point (2, 3, 4) to the line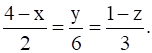 
Also, find the perpendicular distance from the given point to the line.
Given: - Point P(2, 3, 4) and the equation of the line 
Let, PQ be the perpendicular drawn from P to given line whose endpoint/ foot is Q point.
Thus to find Distance PQ we have to first find coordinates of Q
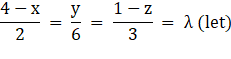
⇒ x = 4 – 2λ, y = 6λ, z = 1 – 3λ
Therefore, coordinates of Q( – 2λ + 4, 6λ, – 3λ + 1)
Now as we know (TIP) ‘if two points A(x1,y1,z1) and B(x2,y2,z2) on a line, then its direction ratios are proportional to (x2 – x1,y2 – y1,z2 – z1)’
Hence
Direction ratio of PQ is
= ( – 2λ + 4 – 2), (6λ – 3), ( – 3λ + 1 – 4)
= ( – 2λ + 2), (6λ – 3), ( – 3λ – 3)
and by comparing with given line equation, direction ratios of the given line are
(hint: denominator terms of line equation)
= ( – 2,6, – 3)
Since PQ is perpendicular to given line, therefore by “condition of perpendicularity.”
a1a2 + b1b2 + c1c2 = 0 ; where a terms and b terms are direction ratio of lines which are perpendicular to each other.
⇒ – 2( – 2λ + 2) + (6)(6λ – 3) – 3( – 3λ – 3) = 0
⇒ 4λ – 4 + 36λ – 18 + 9λ + 9 = 0
⇒ 49λ – 13 = 0
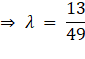
Therefore coordinates of Q
i.e. Foot of perpendicular
By putting the value of λ in Q coordinate equation, we get
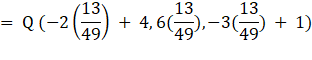
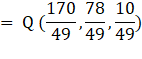
Now,
Distance between PQ
Tip: - Distance between two points A(x1,y1,z1) and B(x2,y2,z2) is given by
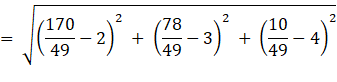
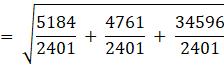
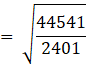
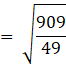
units