The perpendicular from A on side BC of aΔABC intersects BC at D such that DB = 3 CD(see Fig. 6.55). Prove that ![]()
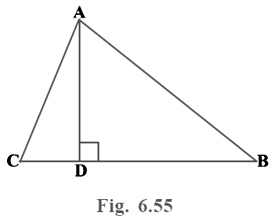
Applying Pythagoras theorem for ΔACD, we obtain
AC2 = AD2 + DC2
AD2 = AC2 – DC2 (i)
Applying Pythagoras theorem in ΔABD, we obtain
AB2 = AD2 + DB2
AD2 = AB2 – DB2 (ii)
From (i) and (ii), we get
AC2 – DC2 = AB2 – DB2 (iii)
It is given that 3DC = DB
Therefore,
DC = ![]() and DB =
and DB = ![]()
Putting these values in (iii), we get
AC2 – ![]() 2 = AB2 –
2 = AB2 – ![]() 2
2
AC2 – ![]() = AB2 –
= AB2 – ![]()
16AC2 – BC2 = 16AB2 – 9BC2
16AB2 – 16AC2 = 8BC2
2AB2 = 2AC2 + BC2
14